Home Donate New Search Gallery How-To Books Links Workshops About Contact
Crop Factor
© 2009 KenRockwell.com
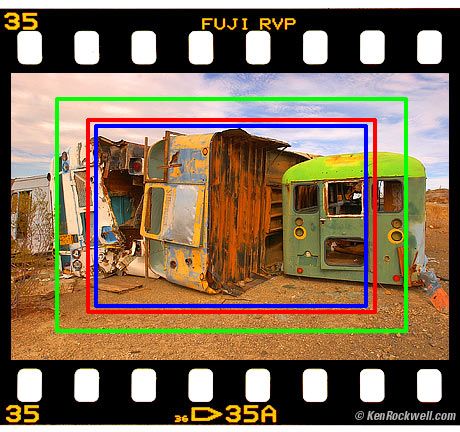
35mm film marked with digital camera sensor sizes. (Green: Canon 1.3x, Red: Nikon DX, Blue: Canon
1.6x. Nikon FX and Canon full-frame are the same size as the image in the film.)
|
I personally buy from Adorama, Amazon, Ritz, B&H, Calumet and J&R. I can't vouch for ads below.
|
Introduction
Most digital camera sensors are smaller than film, so any image you see from those cameras is created from a smaller area than film.
If a photo is made with the same lens, but a smaller sensor, it shows a smaller area.
This is why it's called a crop factor. The smaller sensor is cropping the lens' image compared to a 35mm film frame. Ditto for what you see through the viewfinder.
This is why most digital cameras have smaller viewfinders than 35mm film cameras. In case you've forgotten, go look through your old Canon AE-1 or Nikon F and you'll see a huge viewfinder unlike today's digital SLRs.
When enlarged to the same print or image size, the photo made from the smaller sensor must be enlarged more. This is done automatically. This is why some people call this a magnification factor.
To get this same crop effect, one would have to use an equivalently longer lens on a 35mm film camera.
Nothing changes about the lens; it's simply the amount of the image we use from the back of the lens.
Digital and film SLR lenses are the focal length as marked. They will see a narrower range on a digital camera, and you can estimate the focal length required on a 35mm film camera to see this same, smaller range by multiplying the focal length by a crop factor, usually about 1.5, which depends on the exact sensor size.
Sometimes compact cameras with fixed lenses will list the 35mm equivalent focal length only, since compact cameras have huge crop factors of about 6. A 6mm lens on a compact camera may see the same angle as a 36mm lens on a 35mm film camera.
Examples
The example above shows a 35mm film frame. I've drawn boxes on top of it in the sizes of popular digital cameras. The green box is the sensor size of 1.3x factor cameras, which are the Canon 1D series. The red box is the size of the 1.5x factor sensors in the Nikon digital SLRs. The blue box is the size of the Canon 1.6x consumer cameras' sensors. I didn't draw a box around the 35mm image, even though some Canon cameras (5D and 1Ds) have a big-as film, or "full frame," sensors.
Here are the images you'd get if you stood in the same place and shot with the same lens on these different cameras. These are what's inside each colored box above.

Image from 35mm film or full-frame digital camera.

Image from a 1.3x sensor camera (Canon 1D series).

Image from a 1.5x sensor camera (Nikon DX digital).

Image from a 1.6x sensor camera (Canon consumer digital SLRs).
Calculations
Multiply a lens' focal length by a camera's factor to get the focal length of a lens which, when used on a full-frame or 35mm film camera, gives the same angle of view as that lens does on that digital camera.
A 100mm lens on a 1.5x factor camera shows the same area of view that a 150mm lens would show on a 35mm film or full-frame camera.
I've calculated all these for you for the 1.6x and 1.3x cameras on my Canon 1.6x camera and Canon 1.3x camera pages.
Depth of Field
Basics
The lens isn't actually changing its focal length, so the depth of field remains the same. The image formed by the lens is unchanged regardless of the camera behind it.
If you use a shorter lens to get the same field of view, you get the deeper depth of field of the shorter lens.
This means digital SLRs will have more depth of field when shooting at the same angle (shorter real focal length) than 35mm cameras at the same aperture.
This also explains why compact point and shoot cameras have almost unlimited depth of field. For them a 6mm lens is normal!
You may ignore the Hackers' section below, which explains the factors in more detail and then explains how they all tend to cancel each other. Just shoot and don't worry.
For Hackers
Since there is slightly more effective magnification of the lens' image when viewing the print or file, any defocus will be slightly more visible, thus there will be a little less depth of field.
When calculating depth of field tables, divide your favorite circle of confusion by the factor. For instance, if you use 0.030 mm for 35mm film, use 0.020 mm on Nikon digital (0.030 / 1.5 = 0.020).
If you don't want to calculate your own tables, to get exactly the same depth of field as you did on a film camera, multiply the aperture by the factor. (This does the same thing as using a different circle of confusion for calculations, so don't change both at the same time.) If you get a certain depth of field at f/10 on a 35mm film camera, in the same conditions you'll get the same depth of field at f/16 (1.6 x 10) with the same lens on a 1.6x factor camera. That's about a stop, and there isn't much real change in depth of field with a one-stop change.
This applies even less in reality, because image sharpness is more affected by diffraction as you stop down. Don't worry about it; just shoot.
If you use a shorter lens (a 60mm lens on a 1.6x camera instead of a 100mm lens on a 35mm film camera) then you will get more depth of field at the same aperture.
This is because there are huge changes in depth of field as you change real (optical, not equivalent) focal length. There is a square factor involved, so if you decrease a focal length by 1.4x (going from 28mm to 20mm) you get the same depth of field with an f/number of one half. Per Nikon's depth of field scales, you can get everything from infinity to five feet in focus at f/4 on a 20mm lens, but need f/8 to do it on a 28mm lens. (f/4 x ((28/20)^2) = f/8.)
Personally I consider depth of field tables and scales silly. Depth of field isn't absolute; it depends on your attitude, the magnification of the print, viewing distance and a zillion other things. Depth of field indications are therefore estimates at best, so any attempt to nail them down with any accuracy is futile. They don't mean anything, since they are all drawn up based on arbitrary circles of confusion, and even worse, ignore diffraction. Diffraction is a real issue with small sensors on digital cameras, as you can see at my Sharpness by Aperture page.
Because the sharpest image usually requires an aperture smaller than what a depth of field table says when it suggests a large aperture, and requires a larger aperture when a depth of field table suggests a small aperture, I did all the complex math back in 1991 and developed a system which considers both the effects of defocus (worse at large apertures) and the effects of diffraction (worse at small apertures). See my page on Selecting the Sharpest Aperture.
PLUG top
I support my growing family through this website, as crazy as it might seem.
If you find this as helpful as a book you might have had to buy or a workshop you may have had to take, feel free to help me continue helping everyone.
If you've gotten your gear through one of my links or helped otherwise, you're family. It's great people like you who allow me to keep adding to this site full-time. Thanks!
If you haven't helped yet, please do, and consider helping me with a gift of $5.00.
The biggest help is to use these links to Adorama, Amazon, B&H, Calumet, Ritz and J&R when you get your goodies. It costs you nothing and is a huge help. These places have the best prices and service, which is why I've used them since before this website existed. I recommend them all personally.
Thanks for reading!
Ken
Home Donate New Search Gallery How-To Books Links Workshops About Contact